A craftsman was hired to make a square-shaped stained glass window by the town church. The clergy
specified the size of the window required and the thickness (one inch) and chose the colors. The craftsman
then went back to his shop, and began working on his masterpiece.
Unfortunately the craftsman was not so good at math, and miscalculated the amount of material he would
need. He purchased enough material to cover an area of
508 cubic inches. However, just as he was
ready to make the last glass pane at the very center of the window, he ran out of material leaving a small
square hole shown in black below.
The next day he unveiled his masterpiece to the clergy. When the clergy asked about the hole, he
paused then replied "That's the new style of course!" The question for you is, how many cubic inches is the
hole?
HINT: As you can tell from the image, it is made up of nested squares, rotated 45 degrees as you
work outward. One way to go about the problem is to label one side of each square, starting with the
square hole. Then, express the area of the square in terms of the area of the square surrounding it. For
example, if you label the width/height of the hole as
a and the width/height of the
square surrounding it as
b then
a is the hypotenuse of a triangle with
the other two sides being
b/2.
ANSWER:
4 cubic inches.
EXPLANATION: As you can tell from the image, it is made up of nested squares, rotated 45 degrees as
you work outward. Starting from the hole in the middle and working outwards, label one side of each
square. Then, express the area of the square in terms of the area of the square surrounding it. If you
label the width/height of the hole as
a and the width/height of the square surrounding it
as
b then
a is the hypotenuse of a triangle with the other two sides
being
b/2. Following Pythagoras' theorem:
(b/2)² +
(b/2)² =
a² and ultimately
a² =
b²/2. Now we have the area of the square expressed in terms of the width/height of
the surrounding square (it turns out the area of the inner shape is exactly half the area of the outer
shape). This is no coincidence as you can see here:
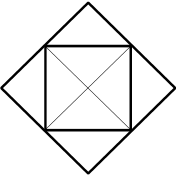
The outer square (diamond) contains eight triangles and the inner square contains four. You can continue
labeling surrounding squares (the last one will be
h) and work outwards until you have
expressed the area of the hole in terms of
h². Ultimately you will arrive at
a² =
h²/128. OR, you can simply apply the pattern (that the
area of each square is half the area of the square surrounding it) and arrive at the same conclusion.
Now you have the total volume of the window if there were no hole (
h²) = 508
(the volume of the material used) plus the volume of the hole which is
h²/128 x 1
(the thickness of the window). This yields
(127/128)h² = 508 and eventually
h² = 512 (the volume of the window if there were no hole). Since the craftsman
used 508 cubic inches of stained glass, that means the volume of the hole is 512 - 508 = 4 cubic inches.
Do you have a
suggestion for this puzzle (e.g. something that should
be mentioned/clarified in the question or solution, bug, typo, etc.)?
